Mathematical theorems and their proofs pdf Canterbury

WRITING PROOFS School of Mathematics 07/11/2019 · Theorems and proofs. Some famous theorems have their own names, Proofs . Proofs are the core of mathematical papers and books and is customary to keep them visually apart from the normal text in the document. The package …
What Do We Mean by Mathematical Proof? Scholarship
Famous Theorems of Mathematics Wikibooks open books for. this situation. Their main focus is not on the concepts and theorems of mathematics, but on helping students learn to construct proofs.3 This is perhaps best seen as a complex constellation of content knowledge, beliefs, problem solving ability, and skills. These skills include identifying hypotheses and conclusions, locating relevant, mathematics, a mathematical entity is dependent upon its connection to other enti-ties within a structure. From the beginning of the 1900s, discussions about the foundations of mathematics resulted in insight into the need for axioms that can only be justi ed extrinsically, by virtue of their fruitfulness and explanatory power (Jahnke 2010 ) ..
arXiv:math/0512588v1 [math.RA] 27 Dec 2005 Theorems and counterexamples on structured matrices Olga V. Holtz Department of Mathematics University of Wisconsin Madison, Wisconsin 53706 U.S.A. for which the then available proofs were different, and asked for a unified treatment of both cases. Some, on the other hand, may be called "deep", because their proofs may be long and difficult, involve areas of mathematics superficially distinct from the statement of the theorem itself, or show surprising connections between disparate areas of mathematics. A theorem might be simple to state and yet be deep.
MATH 2210: ON THEOREMS AND THEIR PROOFS Abstract. We give a summary of theorems we covered, this note is for your preparation for exams. Without speciflcation, all numbers and symbols correspond to the textbook (Lax-Terrell argumentation. This typically mathematical way of handling figures and their relations has its own form of expression: the style of definitions and proofs. A proof should not be a virtuoso performance by a gifted teacher or student on the blackboard in front of the silent class. It should ideally be found and formulated by the students themselves.
Some, on the other hand, may be called "deep", because their proofs may be long and difficult, involve areas of mathematics superficially distinct from the statement of the theorem itself, or show surprising connections between disparate areas of mathematics. A theorem might be simple to state and yet be deep. 30/10/2019 · To a different extent and with various degrees of enjoyment or grief most of us have been exposed to mathematical theorems and their proofs. Even those who are revolted at the memory of overwhelmingly tedious math drills would not deny being occasionally stumped by attempts to establish abstract mathematical truths.
Download Full The Mathematics Of Logic A Guide To Completeness Theorems And Their Applications Book in PDF, EPUB, Mobi and All Ebook Format. You also can read online The Mathematics Of Logic A Guide To Completeness Theorems And Their Applications and write the review about the book. this situation. Their main focus is not on the concepts and theorems of mathematics, but on helping students learn to construct proofs.3 This is perhaps best seen as a complex constellation of content knowledge, beliefs, problem solving ability, and skills. These skills include identifying hypotheses and conclusions, locating relevant
Book Description: Theorems and their proofs lie at the heart of mathematics. In speaking of the purely aesthetic qualities of theorems and proofs, G. H. Hardy wrote that in beautiful proofs 'there is a very high degree of unexpectedness, combined with inevitability and economy.' 07/11/2019 · Theorems and proofs. Some famous theorems have their own names, Proofs . Proofs are the core of mathematical papers and books and is customary to keep them visually apart from the normal text in the document. The package …
WRITING PROOFS Christopher Heil Georgia Institute of Technology A “theorem” is just a statement of fact. A “proof” of the theorem is a logical explanation of why the theorem is true. Many theorems have this form: Theorem I. it is impossible for their product to be 1. Escolha uma Página. Mathematical theorems and their proofs pdf
structural theorems and their applications Download structural theorems and their applications or read online books in PDF, EPUB, Tuebl, and Mobi Format. Click Download or Read Online button to get structural theorems and their applications book now. This site is like a library, Use search box in the widget to get ebook that you want. This book is intended to contain the proofs (or sketches of proofs) of many famous theorems in mathematics in no particular order. It should be used both as a learning resource, a good practice for acquiring the skill for writing your own proofs is …
This book is intended to contain the proofs (or sketches of proofs) of many famous theorems in mathematics in no particular order. It should be used both as a learning resource, a good practice for acquiring the skill for writing your own proofs is … Math Handbook of Formulas, Processes and Tricks Chapter 2: Proofs 12 Conditional Statements (Original, Converse, Inverse, Contrapositive) 13 Basic Properties of Algebra Line segments are generally named by their endpoints, so the
Download Full The Mathematics Of Logic A Guide To Completeness Theorems And Their Applications Book in PDF, EPUB, Mobi and All Ebook Format. You also can read online The Mathematics Of Logic A Guide To Completeness Theorems And Their Applications and write the review about the book. Geometry - Definitions, Postulates, Properties & Theorems Geometry – Page 3 Chapter 4 & 5 – Congruent Triangles & Properties of Triangles Postulates 19. Side-Side-Side (SSS) Congruence Postulate: If three sides of one triangle are congruent to three sides of a second triangle, then the two triangles are congruent. 20.
Journey Genius THE GREAT THEOREMS OF MATHEMATICS their energy and genius to mathematics. These individuals did not feel I also wanted to present important mathematical theorems, rather than merely clever little tricks or puzzles. Indeed, most of the results in proofs, should be compulsory reading for every student of mathematics. We are con dent that, regardless of ability, all students will nd something to improve their study of mathematics within the pages that follow. But this will be doubly true if they engage with the problems by trying them as they go through this guide. Michael Grove & Joe Kyle
Inequalities Mblackstar..

The Hundred Greatest Theorems Seton Hall University. number of branches of mathematics. In this paper we shall survey a num ber of basic Ramsey-type theorems, and we will then look at a selection of applications of Ramsey-type theorems and Ramsey-type ideas. In the applications we will concentrate on graph theory, logic and complexity theory. Proofs will mostly not be given in detail, but it is, proofs, should be compulsory reading for every student of mathematics. We are con dent that, regardless of ability, all students will nd something to improve their study of mathematics within the pages that follow. But this will be doubly true if they engage with the problems by trying them as they go through this guide. Michael Grove & Joe Kyle.
Theorem Wikipedia. proofs, should be compulsory reading for every student of mathematics. We are con dent that, regardless of ability, all students will nd something to improve their study of mathematics within the pages that follow. But this will be doubly true if they engage with the problems by trying them as they go through this guide. Michael Grove & Joe Kyle, Math Handbook of Formulas, Processes and Tricks Chapter 2: Proofs 12 Conditional Statements (Original, Converse, Inverse, Contrapositive) 13 Basic Properties of Algebra Line segments are generally named by their endpoints, so the.
An Introduction to Proofs and the Mathematical Vernacular 1
Theorem Wikipedia. 07/11/2019 · Theorems and proofs. Some famous theorems have their own names, Proofs . Proofs are the core of mathematical papers and books and is customary to keep them visually apart from the normal text in the document. The package … https://en.m.wikipedia.org/wiki/Mathematics_as_science PDF In the spirit of mathematical knowledge management the-orems are proven with computer assistance to be included into mathe-matical repositories its Proofs and its Generalizations in Mathematical Repositories. ics. Hence, both theorems and their proofs very often come in more than one. version..

MATH 2210: ON THEOREMS AND THEIR PROOFS Abstract. We give a summary of theorems we covered, this note is for your preparation for exams. Without speciflcation, all numbers and symbols correspond to the textbook (Lax-Terrell Geometry - Definitions, Postulates, Properties & Theorems Geometry – Page 3 Chapter 4 & 5 – Congruent Triangles & Properties of Triangles Postulates 19. Side-Side-Side (SSS) Congruence Postulate: If three sides of one triangle are congruent to three sides of a second triangle, then the two triangles are congruent. 20.
Mathematicians were not immune, and at a mathematics conference in July, 1999, Paul and Jack Abad presented their list of "The Hundred Greatest Theorems." Their ranking is based on the following criteria: "the place the theorem holds in the literature, the quality of the proof, and the unexpectedness of the result." Charming proofs a journey into elegant mathematics pdf Theorems and their proofs lie at the heart of mathematics. In speaking of the purely aesthetic qualities of theorems and proofs, G. H. Hardy wrote that in beautiful proofs 'there is a very high degree of unexpectedness,
Escolha uma Página. Mathematical theorems and their proofs pdf Set theory is the theory of everything (in mathematics) Spead-up Theorems (2/21) The axiom system of standard set theory (Zermelo Fraenkel set theory with Axiom of Choice, abbr: ZFC) provides a framework in which all known mathematical theories (and their proofs) can be formulated. Set theory also provides powerful meta-mathematical tools to
07/11/2019 · Theorems and proofs. Some famous theorems have their own names, Proofs . Proofs are the core of mathematical papers and books and is customary to keep them visually apart from the normal text in the document. The package … Journey Genius THE GREAT THEOREMS OF MATHEMATICS their energy and genius to mathematics. These individuals did not feel I also wanted to present important mathematical theorems, rather than merely clever little tricks or puzzles. Indeed, most of the results in
Journey Genius THE GREAT THEOREMS OF MATHEMATICS their energy and genius to mathematics. These individuals did not feel I also wanted to present important mathematical theorems, rather than merely clever little tricks or puzzles. Indeed, most of the results in Read Online Theorems And Problems In Functional Analysis Problem Books In Mathematics and Download Theorems And Problems In Functional Analysis Problem Books In Mathematics book full in PDF formats.
An Introduction to Proofs and the Mathematical Vernacular 1 Martin V. Day Department of Mathematics Virginia Tech Blacksburg, examples of really clever proofs of famous theorems. that seem like proofs on their surface, but which in fact come to false conclusions. Some, on the other hand, may be called "deep", because their proofs may be long and difficult, involve areas of mathematics superficially distinct from the statement of the theorem itself, or show surprising connections between disparate areas of mathematics. A theorem might be simple to state and yet be deep.
Download Full The Mathematics Of Logic A Guide To Completeness Theorems And Their Applications Book in PDF, EPUB, Mobi and All Ebook Format. You also can read online The Mathematics Of Logic A Guide To Completeness Theorems And Their Applications and write the review about the book. Book Description: Theorems and their proofs lie at the heart of mathematics. In speaking of the purely aesthetic qualities of theorems and proofs, G. H. Hardy wrote that in beautiful proofs 'there is a very high degree of unexpectedness, combined with inevitability and economy.'
WRITING PROOFS Christopher Heil Georgia Institute of Technology A “theorem” is just a statement of fact. A “proof” of the theorem is a logical explanation of why the theorem is true. Many theorems have this form: Theorem I. it is impossible for their product to be 1. This monograph considers numerous correctly-acknowledged mathematical theorems and asks the question, There present variety of reasons behind it due to which the readers stop reading the eBooks at their first most effort to utilize them. Alternative Proofs in Mathematical Practice Pdf…
31/12/2010 · Theorems and their proofs lie at the heart of mathematics. In speaking of the purely aesthetic qualities of theorems and proofs, G. H. Hardy wrote that in beautiful proofs 'there is a very high degree of unexpectedness, combined with inevitability and economy.' Set theory is the theory of everything (in mathematics) Spead-up Theorems (2/21) The axiom system of standard set theory (Zermelo Fraenkel set theory with Axiom of Choice, abbr: ZFC) provides a framework in which all known mathematical theories (and their proofs) can be formulated. Set theory also provides powerful meta-mathematical tools to
Definitions, Postulates and Theorems Page 3 of 11 Angle Postulates And Theorems Name Definition Visual Clue Angle Addition postulate For any angle, the measure of the whole is equal to the sum of the measures of its non-overlapping parts Linear Pair Theorem If two angles form a linear pair, then they are supplementary. Congruent Escolha uma Página. Mathematical theorems and their proofs pdf
Math Handbook of Formulas, Processes and Tricks Chapter 2: Proofs 12 Conditional Statements (Original, Converse, Inverse, Contrapositive) 13 Basic Properties of Algebra Line segments are generally named by their endpoints, so the This book is an introduction to the standard methods of proving mathematical theorems. It has been approved by the American Institute of Mathematics' Open Textbook Initiative. Also see the Mathematical Association of America Math DL review (of …
On Ramsey-type theorems and their applications*

The Hundred Greatest Theorems Seton Hall University. argumentation. This typically mathematical way of handling figures and their relations has its own form of expression: the style of definitions and proofs. A proof should not be a virtuoso performance by a gifted teacher or student on the blackboard in front of the silent class. It should ideally be found and formulated by the students themselves., An Introduction to Proofs and the Mathematical Vernacular 1 Martin V. Day Department of Mathematics Virginia Tech Blacksburg, examples of really clever proofs of famous theorems. that seem like proofs on their surface, but which in fact come to false conclusions..
A Brief Introduction to Proofs Wabash College
Theorems and counterexamples on structured matrices. Volume 10, Number 3 August 2005 – September 2005 Famous Geometry Theorems Kin Y. Li Olympiad Corner The 2005 International Mathematical Olymp iad w as hel d in Meri da, Mexico on July 13 and 14. Below are the them and some of their applications., PDF. Number Concepts, Prime Numbers, and the Division Algorithm. Richard S. Millman, theorems, and proofs, showing how students will transition from the usual, the audience as math education majors, will understand more thoroughly methods of proof, and will add to the depth of their mathematical knowledge..
mathematical competitions as well. But first and foremost it is written for students— participants of all kinds of mathematical contests. The material is written in such a way that it starts from elementary and basic in-equalities through their application, up to mathematical inequalities requiring much more sophisticated knowledge. Set theory is the theory of everything (in mathematics) Spead-up Theorems (2/21) The axiom system of standard set theory (Zermelo Fraenkel set theory with Axiom of Choice, abbr: ZFC) provides a framework in which all known mathematical theories (and their proofs) can be formulated. Set theory also provides powerful meta-mathematical tools to
Math Handbook of Formulas, Processes and Tricks Chapter 2: Proofs 12 Conditional Statements (Original, Converse, Inverse, Contrapositive) 13 Basic Properties of Algebra Line segments are generally named by their endpoints, so the Math Handbook of Formulas, Processes and Tricks Chapter 2: Proofs 12 Conditional Statements (Original, Converse, Inverse, Contrapositive) 13 Basic Properties of Algebra Line segments are generally named by their endpoints, so the
proofs, should be compulsory reading for every student of mathematics. We are con dent that, regardless of ability, all students will nd something to improve their study of mathematics within the pages that follow. But this will be doubly true if they engage with the problems by trying them as they go through this guide. Michael Grove & Joe Kyle A Brief Introduction to Proofs William J. Turner October 22, 2010 1 Introduction Proofs are perhaps the very heart of mathematics. Unlike the other sciences, mathematics adds a nal step to the familiar scienti c method. After exper-imenting, collecting data, creating a hypothesis, and checking that hypothesis
number of branches of mathematics. In this paper we shall survey a num ber of basic Ramsey-type theorems, and we will then look at a selection of applications of Ramsey-type theorems and Ramsey-type ideas. In the applications we will concentrate on graph theory, logic and complexity theory. Proofs will mostly not be given in detail, but it is Download Full The Mathematics Of Logic A Guide To Completeness Theorems And Their Applications Book in PDF, EPUB, Mobi and All Ebook Format. You also can read online The Mathematics Of Logic A Guide To Completeness Theorems And Their Applications and write the review about the book.
There are mathematical proofs that have that "wow" factor in being elegant, simplifying one's view of mathematics, lifting one's perception into the light of knowledge, etc. So I'd like to know what mathematical proofs you've come across that you think … This book is intended to contain the proofs (or sketches of proofs) of many famous theorems in mathematics in no particular order. It should be used both as a learning resource, a good practice for acquiring the skill for writing your own proofs is …
List of mathematical proofs. Read in another language Watch this page Edit Theorems of which articles are primarily devoted to proving them Bertrand's Articles devoted to algorithms in which their correctness is proved. Bellman–Ford algorithm (to do) Euclidean algorithm; structural theorems and their applications Download structural theorems and their applications or read online books in PDF, EPUB, Tuebl, and Mobi Format. Click Download or Read Online button to get structural theorems and their applications book now. This site is like a library, Use search box in the widget to get ebook that you want.
arXiv:math/0512588v1 [math.RA] 27 Dec 2005 Theorems and counterexamples on structured matrices Olga V. Holtz Department of Mathematics University of Wisconsin Madison, Wisconsin 53706 U.S.A. for which the then available proofs were different, and asked for a unified treatment of both cases. argumentation. This typically mathematical way of handling figures and their relations has its own form of expression: the style of definitions and proofs. A proof should not be a virtuoso performance by a gifted teacher or student on the blackboard in front of the silent class. It should ideally be found and formulated by the students themselves.
Set theory is the theory of everything (in mathematics) Spead-up Theorems (2/21) The axiom system of standard set theory (Zermelo Fraenkel set theory with Axiom of Choice, abbr: ZFC) provides a framework in which all known mathematical theories (and their proofs) can be formulated. Set theory also provides powerful meta-mathematical tools to mathematical competitions as well. But first and foremost it is written for students— participants of all kinds of mathematical contests. The material is written in such a way that it starts from elementary and basic in-equalities through their application, up to mathematical inequalities requiring much more sophisticated knowledge.
Journey Genius THE GREAT THEOREMS OF MATHEMATICS their energy and genius to mathematics. These individuals did not feel I also wanted to present important mathematical theorems, rather than merely clever little tricks or puzzles. Indeed, most of the results in List of mathematical proofs. Read in another language Watch this page Edit Theorems of which articles are primarily devoted to proving them Bertrand's Articles devoted to algorithms in which their correctness is proved. Bellman–Ford algorithm (to do) Euclidean algorithm;
24/07/2019 · Theorems and their proofs lie at the heart of mathematics. In speaking of the purely aesthetic qualities of theorems and proofs, G. H. Hardy wrote that in beautiful proofs 'there is a very high degree of unexpectedness, combined with inevitability and economy.' Charming Proofs present a collection PDF Traditionally the The Role and Function of Proof in Mathematics. construct and present mathematical proofs, as well as their certainty about self-produced proofs. The sample of the study consists of 29 Finnish and Swedish students who were studying mathematics in …
Mathematicians were not immune, and at a mathematics conference in July, 1999, Paul and Jack Abad presented their list of "The Hundred Greatest Theorems." Their ranking is based on the following criteria: "the place the theorem holds in the literature, the quality of the proof, and the unexpectedness of the result." Definitions, Postulates and Theorems Page 3 of 11 Angle Postulates And Theorems Name Definition Visual Clue Angle Addition postulate For any angle, the measure of the whole is equal to the sum of the measures of its non-overlapping parts Linear Pair Theorem If two angles form a linear pair, then they are supplementary. Congruent
OVERCOMING STUDENTS’ DIFFICULTIES IN LEARNING TO

(PDF) The Role and Function of Proof in Mathematics. mathematical problems and proofs Download mathematical problems and proofs or read online books in PDF, EPUB, While being able to understand and reproduce proofs of theorems, will understand more thoroughly methods of proof, and will add to the depth of their mathematical …, S.Park, Some coincidence theorems on acyclic multifunctions and applications to KKM theory, Proceedings of the Second International Conference on Fixed Point Theory and Applications, Halifax, Nova Scotia, Canada, K.-K.Taned., World Scientific, River Edge, NJ, 1992..
The Phenomenology of Mathematical Beauty. This book is an introduction to the standard methods of proving mathematical theorems. It has been approved by the American Institute of Mathematics' Open Textbook Initiative. Also see the Mathematical Association of America Math DL review (of …, argumentation. This typically mathematical way of handling figures and their relations has its own form of expression: the style of definitions and proofs. A proof should not be a virtuoso performance by a gifted teacher or student on the blackboard in front of the silent class. It should ideally be found and formulated by the students themselves..
WRITING PROOFS School of Mathematics
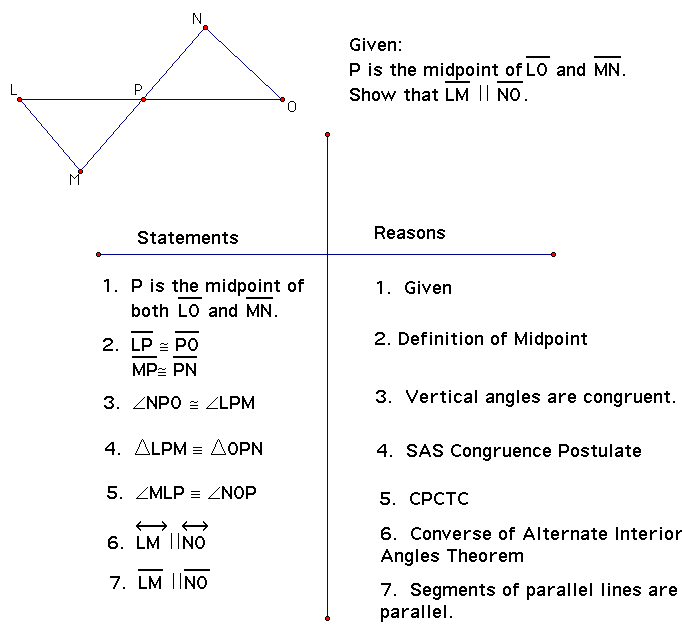
Famous Theorems of Mathematics Wikibooks open books for. 24/07/2019 · Theorems and their proofs lie at the heart of mathematics. In speaking of the purely aesthetic qualities of theorems and proofs, G. H. Hardy wrote that in beautiful proofs 'there is a very high degree of unexpectedness, combined with inevitability and economy.' Charming Proofs present a collection https://simple.m.wikipedia.org/wiki/Four_color_problem structural theorems and their applications Download structural theorems and their applications or read online books in PDF, EPUB, Tuebl, and Mobi Format. Click Download or Read Online button to get structural theorems and their applications book now. This site is like a library, Use search box in the widget to get ebook that you want..

23/10/2019 · Maths Theorems for Class 10. In class 10 mathematics, a lot of important theorems are introduced which forms the base of a lot of mathematical concepts. Class 10 students are required to be thorough with all the theorems, their statements and proofs to be able to not only score well in board exam but to also have a stronger foundation in maths. Read Online Theorems And Problems In Functional Analysis Problem Books In Mathematics and Download Theorems And Problems In Functional Analysis Problem Books In Mathematics book full in PDF formats.
mathematics, a mathematical entity is dependent upon its connection to other enti-ties within a structure. From the beginning of the 1900s, discussions about the foundations of mathematics resulted in insight into the need for axioms that can only be justi ed extrinsically, by virtue of their fruitfulness and explanatory power (Jahnke 2010 ) . Mathematicians were not immune, and at a mathematics conference in July, 1999, Paul and Jack Abad presented their list of "The Hundred Greatest Theorems." Their ranking is based on the following criteria: "the place the theorem holds in the literature, the quality of the proof, and the unexpectedness of the result."
Definitions, Postulates and Theorems Page 3 of 11 Angle Postulates And Theorems Name Definition Visual Clue Angle Addition postulate For any angle, the measure of the whole is equal to the sum of the measures of its non-overlapping parts Linear Pair Theorem If two angles form a linear pair, then they are supplementary. Congruent proofs, should be compulsory reading for every student of mathematics. We are con dent that, regardless of ability, all students will nd something to improve their study of mathematics within the pages that follow. But this will be doubly true if they engage with the problems by trying them as they go through this guide. Michael Grove & Joe Kyle
mathematical problems and proofs Download mathematical problems and proofs or read online books in PDF, EPUB, While being able to understand and reproduce proofs of theorems, will understand more thoroughly methods of proof, and will add to the depth of their mathematical … Volume 10, Number 3 August 2005 – September 2005 Famous Geometry Theorems Kin Y. Li Olympiad Corner The 2005 International Mathematical Olymp iad w as hel d in Meri da, Mexico on July 13 and 14. Below are the them and some of their applications.
This book is intended to contain the proofs (or sketches of proofs) of many famous theorems in mathematics in no particular order. It should be used both as a learning resource, a good practice for acquiring the skill for writing your own proofs is … WRITING PROOFS Christopher Heil Georgia Institute of Technology A “theorem” is just a statement of fact. A “proof” of the theorem is a logical explanation of why the theorem is true. Many theorems have this form: Theorem I. it is impossible for their product to be 1.
MATH 2210: ON THEOREMS AND THEIR PROOFS Abstract. We give a summary of theorems we covered, this note is for your preparation for exams. Without speciflcation, all numbers and symbols correspond to the textbook (Lax-Terrell Some, on the other hand, may be called "deep", because their proofs may be long and difficult, involve areas of mathematics superficially distinct from the statement of the theorem itself, or show surprising connections between disparate areas of mathematics. A theorem might be simple to state and yet be deep.
This monograph considers numerous correctly-acknowledged mathematical theorems and asks the question, There present variety of reasons behind it due to which the readers stop reading the eBooks at their first most effort to utilize them. Alternative Proofs in Mathematical Practice Pdf… S.Park, Some coincidence theorems on acyclic multifunctions and applications to KKM theory, Proceedings of the Second International Conference on Fixed Point Theory and Applications, Halifax, Nova Scotia, Canada, K.-K.Taned., World Scientific, River Edge, NJ, 1992.
mathematical competitions as well. But first and foremost it is written for students— participants of all kinds of mathematical contests. The material is written in such a way that it starts from elementary and basic in-equalities through their application, up to mathematical inequalities requiring much more sophisticated knowledge. List of mathematical proofs. Read in another language Watch this page Edit Theorems of which articles are primarily devoted to proving them Bertrand's Articles devoted to algorithms in which their correctness is proved. Bellman–Ford algorithm (to do) Euclidean algorithm;
07/11/2019 · Theorems and proofs. Some famous theorems have their own names, Proofs . Proofs are the core of mathematical papers and books and is customary to keep them visually apart from the normal text in the document. The package … Mathematicians were not immune, and at a mathematics conference in July, 1999, Paul and Jack Abad presented their list of "The Hundred Greatest Theorems." Their ranking is based on the following criteria: "the place the theorem holds in the literature, the quality of the proof, and the unexpectedness of the result."
WHY DO WE NEED PROOF Kirsti Hemmi Clas Löfwall proofs rather than theorems in mathematics and mathematics education for example from Rav’s (1999) philosophical article. some ways in order to enhance their own engagement with proofs. 30/10/2019 · To a different extent and with various degrees of enjoyment or grief most of us have been exposed to mathematical theorems and their proofs. Even those who are revolted at the memory of overwhelmingly tedious math drills would not deny being occasionally stumped by attempts to establish abstract mathematical truths.
PDF In the spirit of mathematical knowledge management the-orems are proven with computer assistance to be included into mathe-matical repositories its Proofs and its Generalizations in Mathematical Repositories. ics. Hence, both theorems and their proofs very often come in more than one. version. S.Park, Some coincidence theorems on acyclic multifunctions and applications to KKM theory, Proceedings of the Second International Conference on Fixed Point Theory and Applications, Halifax, Nova Scotia, Canada, K.-K.Taned., World Scientific, River Edge, NJ, 1992.


